Strengths and limitations of the SSCHA

how dows it compare with other approaches?

by Lorenzo Monacelli

Self-Consistent Harmonic Approximation

  • A method to compute the Free energy of the system
    • $$ F = \min_{\hat \rho}\left\{ \braket{H}_{\hat \rho} - T S[\hat \rho]\right\} $$
  • Restrict $\hat\rho$ to solutions of harmonic $\hat{\mathcal H}_{\mathcal{R}, \Phi}$
    • $$ \hat\rho_{\mathcal{R},\Phi} = \frac{e^{-\beta \hat{\mathcal{H}}_{\mathcal{R}, \Phi}}}{\mathcal{Z}} $$

    Self-Consistent Harmonic Approximation

  • A method to compute the Free energy of the system
    • $$ F = \min_{\hat \rho}\left\{ \braket{H}_{\hat \rho} - T S[\hat \rho]\right\} $$
  • Restrict $\hat\rho$ to solutions of harmonic $\hat{\mathcal H}_{\mathcal{R}, \Phi}$
    • $$ \hat{\mathcal{H}}_{\mathcal{R}, \Phi} = \sum_\alpha \frac{ {{\hat P}_\alpha}^2}{2m_\alpha} +\underbrace{\sum_{\alpha\beta}(\hat R_\alpha - {\color{blue}\mathcal{R}_\alpha}) {\color{darkgreen}\Phi_{\alpha\beta}}(\hat R_\beta - {\color{blue}\mathcal{R}_\beta})}_{\mathcal V(R)} $$
  • Minimize $F$ with respect to $\mathcal{R}$ and $\Phi$ $$F \le F[\hat\rho_{\mathcal R, \Phi}] = F_{\mathcal H} + \left< V(R) - \mathcal V(R) \right>_{\hat\rho_{\mathcal R, \Phi}}$$

    • Self-Consistent Harmonic Approximation

  • Minimize $F$ with respect to $\mathcal{R}$ and $\Phi$ $$F \le F[\hat\rho_{\mathcal R, \Phi}] = F_{\mathcal H} + \left< V(R) - \mathcal V(R) \right>_{\hat\rho_{\mathcal R, \Phi}}$$

    • SCHA is a first-principles theory

    • $\Phi$ are not the phonons!
    • Phase-stability through linear response theory
      $$ \frac{d^2 F}{d{\mathcal R}_a d{\mathcal R}_b} = \Phi_{ab} + \stackrel{(3)}{\Phi}_{acd}\Lambda_{cdef}\stackrel{(3)}{\Phi}_{efb} + \ldots $$
    • Dynamics solves a time-dependent Schrödinger equation:
      $$ i\frac{d}{dt} \hat \rho(t) = \left[\hat{\mathcal H}[\hat\rho(t)], \hat\rho(t)\right] $$
    • Phonons are dynamical perturbations
      $$ G_{ab}(t) =\sqrt{m_a m_b}\braket{\left[R_a(t) - {\mathcal R}_a\right] \left[R_b(0) - {\mathcal R}_b\right]} $$

    Strengths of SSCHA

    • Exploit symmetries.
      $S^\dagger \Phi S = \Phi \;\;\;\; S\mathcal R = \mathcal R$
    • Analytic expression for the entropy.
      $$ F = F_{\mathcal H} + \left< V(R) - \mathcal V(R)\right> $$
    • Interpolation on q-mesh: simulate infinite supercells.
    • Rigorous linear-response properties:

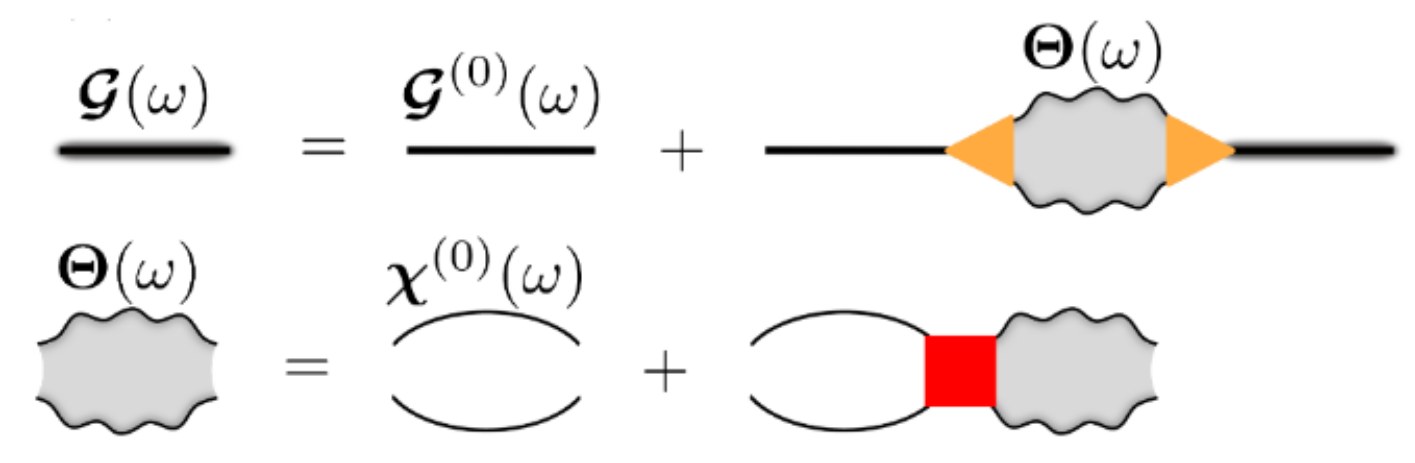
    Weaknesses of SSCHA

    • The probability distribution is a Gaussian.
      • Fails in systems where atoms displace significantly.
      • Ionic diffusion
      • Ionic movements deviating from straight lines
    • Bad scaling in absence of symmetries

    What we cannot do with the SSCHA?

    • Liquids
    • Rotating molecules
    • Glasses

    Comparison with different methods

    SSCHA

    • Quantum zero-point motion
    • Symmetries
    • Space group constraint
    • Analytic free energy
    • Approximated distribution
    • Dynamical Properties
    • $N^3$ scaling

    Molecular Dynamics

    • Classical sampling
    • No symmetries
    • No space group constraint
    • No entropy
    • Exact distribution
    • Dynamical Properties
    • Linear scaling

    Comparison with different methods

    SSCHA

    • Quantum zero-point motion
    • Symmetries
    • Space group constraint
    • Analytic free energy
    • Approximated distribution
    • Dynamical Properties
    • $N^3$ scaling

    Path-Integral Molecular dynamics

    • Quantum sampling
    • No symmetries
    • No space group constraint
    • No entropy
    • Exact distribution
    • No dynamical properties
    • $PN$ linear scaling

    Use the right tool at the right time

    • Is $T \lesssim \frac{\hbar\omega_\text{max}}{k_b}$?
    • Phase-diagram?
    • Dynamical properties?
    • Liquid, amorphous, rotations?
    • Anharmonicity and high T?
    • PIMD, SSCHA, Harmonic
    • SSCHA, Harmonic
    • SSCHA, MD
    • PIMD, MD
    • PIMD, MD, SSCHA

    Alternatives to the SSCHA

    Alternatives to the SSCHA

    • Self-consistent ab initio lattice dynamics (SCAILD)
    • Self-consistent phonons (SCP)
    • Temperature-dependent effective potential (TDEP)
    • Many others ....

    Alternatives to the SSCHA

    The name Self-Consistent Harmonic comes from the equations $$ \Phi_{ab} = \left< \frac{\partial^2V}{\partial R_a \partial R_b} \right>_{\rho_{{\mathcal R}, \Phi}} \qquad 0 = \left < \frac{\partial V}{\partial R_a} \right>_{\rho_{{\mathcal R}, \Phi}} $$ that are solved self-consistently
    • SCAILD and SCP solve the same equations
    • TDEP computes $\Phi_{ab}$ averaging over MD trajectories

    Self-Consistent Ab Initio Lattice Dynamics (SCAILD)

    • Displace atoms along the polarization vectors of $\Phi_{ab}$ $$ R_a^\mu = \mathcal{R}_a + \sum_b e_\mu^b \sqrt{\frac{\hbar\left(2n_\mu + 1\right)}{2\omega_\mu m_b}} $$
    • Compute the ab-initio force on the displaced atoms.
    • Compute the new $\Phi_{ab}$ from the forces $$ f_a(R_a) = -\sum_b \Phi_{ab} (R_b - \mathcal{R}_b) $$
    Souvatzis et al, Phys. Rev. Lett. 100, 095901 (2008)

    Self-Consistent Ab Initio Lattice Dynamics (SCAILD)

    but...

    • This would coincide with the SSCHA if:
      • The lattice vectors $\mathcal R$ do not change
      • The polarization vectors of $\Phi_{ab}$ do not change
    • The free energy contains only the harmonic part: $$ F_{\text{scaild}} \neq F_{\text{sscha}} $$ $$ F_{\text{scaild}} = F_{\mathcal H} + V({\mathcal R}) \qquad F_{\text{sscha}} = F_{\mathcal H} + \braket{V - \mathcal V}_{\rho_{\mathcal R, \Phi}} $$
    • SCAILD is not variational!
    Souvatzis et al, Phys. Rev. Lett. 100, 095901 (2008)

    Temperature Dependent Energy Potential (TDEP)

    • Model the potential as a Taylor expansion with temperature dependent coefficients fitted from AIMD trajectories
      $$ {\mathcal V}({R}, T) = \sum_{ab} \frac{1}{2} \stackrel{(2)}{\Phi}_{ab}(T) (R_a - \mathcal{R}_a) (R_b - \mathcal{R}_b) + $$
      $$ + \sum_{abc} \frac{1}{6} \stackrel{(3)}{\Phi}_{abc}(T) (R_a - \mathcal{R}_a) (R_b - \mathcal{R}_b) (R_c - \mathcal{R}_c) + \dots $$
    • The free energy is evaluated from the harmonic part as: $$ F_{\text{tdep}} = F_{\Phi(T)} + \braket{V - \mathcal V}_{\rho_{\text{MD}}} $$
    • TDEP is not variational!
    Hellman et al, Phys. Rev. B 84, 180301(R) (2011)

    The auxiliary force constant matrix $\Phi(T)$

    • $\Phi(T)$ have no physical meaning.
    • It does not indicate the phase stability. $$ \frac{d^2F}{d{\mathcal R}_a d{\mathcal R}_b} \neq \Phi $$
    • It does not describe the anharmonic phonon energy.
    • The spectral function is not physical.
    • In TDEP, $\Phi$ depends on the order $n$ of the fit of the potential.
      • $\Phi$ is the harmonic force constant matrix if $n\rightarrow\infty$ .

    Self-Consistent Phonons (SCP)

    • Model the potential as a Taylor expansion
      $$ V({R}) = V(\mathcal{R}) + \sum_{ab} \frac{1}{2} \stackrel{(2)}{\Phi}_{ab} (R_a - \mathcal{R}_a) (R_b - \mathcal{R}_b) + $$
      $$ + \sum_{abc} \frac{1}{6} \stackrel{(3)}{\Phi}_{abc} (R_a - \mathcal{R}_a) (R_b - \mathcal{R}_b) (R_c - \mathcal{R}_c) + \dots $$
    • Compressed sensing technique to fit $\stackrel{(2)}{\Phi}$, $\stackrel{(3)}{\Phi}$ and $\stackrel{(4)}{\Phi}$, ...
    • The SSCHA integrals can be solved analytically $$ \Phi_{ab} = \left< \frac{\partial^2 V}{\partial R_a \partial R_b} \right>_{\rho_{{\mathcal R}, \Phi}} \qquad 0 = \left < \frac{\partial V}{\partial R_a} \right>_{\rho_{{\mathcal R}, \Phi}} $$
    Tadano et al, Phys. Rev. B 92, 054301 (2015)

    Self-Consistent Phonons (SCP)

    And

    Temperature Dependent Effective Potential (TDEP)

    • Model the potential as a Taylor expansion
      $$ V({R}) = V(\mathcal{R}) + \sum_{ab} \frac{1}{2} \stackrel{(2)}{\Phi}_{ab} (R_a - \mathcal{R}_a) (R_b - \mathcal{R}_b) + $$
      $$ + \sum_{abc} \frac{1}{6} \stackrel{(3)}{\Phi}_{abc} (R_a - \mathcal{R}_a) (R_b - \mathcal{R}_b) (R_c - \mathcal{R}_c) + \dots $$
    • But in TDEP the force-constants are temperature dependent
      $$ \stackrel{(2)}{\Phi}(T) \qquad \stackrel{(3)}{\Phi}(T) \qquad \stackrel{(4)}{\Phi}(T) \qquad \dots $$
    Tadano et al, Phys. Rev. B 92, 054301 (2015)

    Comparison

    SSCHA SCAILD SCP
    TDEP
    Not Empirical ✓ ✗ ✓ ✗
    Variational ✓ ✗ ✗ ✗
    Computationally cheap ✗ ✓ ✓ ✗
    Relax lattice ✓ ✗ ✓ ✓
    Phase stability ✓ ✗ ✓ ✗
    Phase diagram ✓ ✓ ✓ ✓
    Quantum effects ✓ ✓ ✓ ✗

    Comparison

    SSCHA SCAILD SCP
    TDEP(SCP)
    Not Empirical ✓ ✗ ✓ ✓
    Variational ✓ ✗ ✗ ✗
    Computationally cheap ✗ ✓ ✓ ✗
    Relax lattice ✓ ✗ ✓ ✓
    Phase stability ✓ ✗ ✓ ✓
    Phase diagram ✓ ✓ ✓ ✓
    Quantum effects ✓ ✓ ✓ ✗